Thread: Quite an Unusual one
Threaded View
-
08-12-2014 #11
We can calculate a decent approximation to the inertia of all the parts in the system using this formula for the moment of inertia of a cylinder:
.
Just break down the parts into cylinders and add them all up - e.g. for the ballnut we can consider it as three cylinders - the flange, the smaller diameter bit and the hole.
To find the equivalent inertia of the 120kg gantry, you need to consider the effect of the ballscrew, so this formula is required (where L is the pitch of the screw):
.
The last thing to consider is the effect of the drive ratio - in your case 20/30. This causes the inertia of the parts on the driven shaft (the nut etc) to be scaled by this factor squared. The drive shaft inertia (i.e. motor + 20T pulley) is left as is and we work out the equivalent inertia as if everything was placed on the drive shaft.The total inertia of the system is therefore:
.
Putting all that together (see calculations attached) I get the following values for the total inertia of the system. I've had to guess the dimensions a bit and I don't know the inertia of the motor, so feel free to double check and make it more accurate if you wish.
motor pulley:nut pulley = inertia:
steel:steel = 4.54e-4 kg-m^2
steel:alu = 4.31e-4 kg-m^2
alu:steel = 4.42e-4 kg-m^2
alu:alu = 4.19e-4 kg-m^2
(For interest, equivalent inertia of the 120kg gantry is 1.36e-4 kg-m^2 - about the same as just the RM2510 ballnut!)
So as you can see, in your case the material you select for the pulleys doesn't make too much difference - even with both pulleys made from aluminium the gain is less than 10%. Lets check your not really close to the motor torque rating, else those few % might be valuable. The motor is rated for 400W at 3000rpm, so the torque rating is .. Torque is the product of angular acceleration and inertia, so if we say you want 3m/s^2 acceleration (reasonably snappy for a machine this size) then you need 20/30*2*pi*a/L=1257 rad/s^2 acceleration so using the worst case (steel pulleys), 1257*4.54e-4=0.57Nm. Add to that the frictional losses using this and you're still well below the continuous rating of the motor (let alone the peak), so I'd stick with steel pulleys as they will last longer. Just noticed I momentarily forgot you have two motors, so the required torque is actually even lower...
The other thing to check is the inertia ratio of the system (I.total/I.motor), to make sure it is within the ratings of the servo drive system otherwise the servos may not be able to control the machine stably. The motor is probably around 4e-5 kg-m^2, so your inertia ratio is around 10 at the moment. Do check what the servo system you have(?) can tolerate as that's a bit high. If it's outside the recommended range you can change the pulley sizes to get a better match.
-
The Following 2 Users Say Thank You to Jonathan For This Useful Post:
Thread Information
Users Browsing this Thread
There are currently 1 users browsing this thread. (0 members and 1 guests)










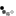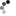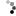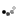
 Reply With Quote
Reply With Quote
Bookmarks